niels bohr emission spectrum experiment

Development of Current Atomic Theory

Emission Spectrum of Hydrogen

When an electric current is passed through a glass tube that contains hydrogen gas at low pressure the tube gives off blue light. When this light is passed through a prism (as shown in the figure below), four narrow bands of bright light are observed against a black background.

These narrow bands have the characteristic wavelengths and colors shown in the table below.

Four more series of lines were discovered in the emission spectrum of hydrogen by searching the infrared spectrum at longer wave-lengths and the ultraviolet spectrum at shorter wavelengths. Each of these lines fits the same general equation, where n 1 and n 2 are integers and R H is 1.09678 x 10 -2 nm -1 .

Explanation of the Emission Spectrum

Max Planck presented a theoretical explanation of the spectrum of radiation emitted by an object that glows when heated. He argued that the walls of a glowing solid could be imagined to contain a series of resonators that oscillated at different frequencies. These resonators gain energy in the form of heat from the walls of the object and lose energy in the form of electromagnetic radiation. The energy of these resonators at any moment is proportional to the frequency with which they oscillate.

To fit the observed spectrum, Planck had to assume that the energy of these oscillators could take on only a limited number of values. In other words, the spectrum of energies for these oscillators was no longer continuous. Because the number of values of the energy of these oscillators is limited, they are theoretically "countable." The energy of the oscillators in this system is therefore said to be quantized . Planck introduced the notion of quantization to explain how light was emitted.

Albert Einstein extended Planck's work to the light that had been emitted. At a time when everyone agreed that light was a wave (and therefore continuous), Einstein suggested that it behaved as if it was a stream of small bundles, or packets, of energy. In other words, light was also quantized. Einstein's model was based on two assumptions. First, he assumed that light was composed of photons , which are small, discrete bundles of energy. Second, he assumed that the energy of a photon is proportional to its frequency.

In this equation, h is a constant known as Planck's constant, which is equal to 6.626 x 10 -34 J-s.

Example: Let's calculate the energy of a single photon of red light with a wavelength of 700.0 nm and the energy of a mole of these photons.

Red light with a wavelength of 700.0 nm has a frequency of 4.283 x 10 14 s -1 . Substituting this frequency into the Planck-Einstein equation gives the following result.

A single photon of red light carries an insignificant amount of energy. But a mole of these photons carries about 171,000 joules of energy, or 171 kJ/mol.

Absorption of a mole of photons of red light would therefore provide enough energy to raise the temperature of a liter of water by more than 40 o C.

The fact that hydrogen atoms emit or absorb radiation at a limited number of frequencies implies that these atoms can only absorb radiation with certain energies. This suggests that there are only a limited number of energy levels within the hydrogen atom. These energy levels are countable. The energy levels of the hydrogen atom are quantized.

The Bohr Model of the Atom

• The electron in a hydrogen atom travels around the nucleus in a circular orbit.
• The energy of the electron in an orbit is proportional to its distance from the nucleus. The further the electron is from the nucleus, the more energy it has.
• Only a limited number of orbits with certain energies are allowed. In other words, the orbits are quantized.
• The only orbits that are allowed are those for which the angular momentum of the electron is an integral multiple of Planck's constant divided by 2p.
• Light is absorbed when an electron jumps to a higher energy orbit and emitted when an electron falls into a lower energy orbit.
• The energy of the light emitted or absorbed is exactly equal to the difference between the energies of the orbits.

Some of the key elements of this hypothesis are illustrated in the figure below.

Three points deserve particular attention. First, Bohr recognized that his first assumption violates the principles of classical mechanics. But he knew that it was impossible to explain the spectrum of the hydrogen atom within the limits of classical physics. He was therefore willing to assume that one or more of the principles from classical physics might not be valid on the atomic scale.

Second, he assumed there are only a limited number of orbits in which the electron can reside. He based this assumption on the fact that there are only a limited number of lines in the spectrum of the hydrogen atom and his belief that these lines were the result of light being emitted or absorbed as an electron moved from one orbit to another in the atom.

Finally, Bohr restricted the number of orbits on the hydrogen atom by limiting the allowed values of the angular momentum of the electron. Any object moving along a straight line has a momentum equal to the product of its mass ( m ) times the velocity ( v ) with which it moves. An object moving in a circular orbit has an angular momentum equal to its mass ( m ) times the velocity ( v ) times the radius of the orbit ( r ). Bohr assumed that the angular momentum of the electron can take on only certain values, equal to an integer times Planck's constant divided by 2p.

Bohr then used classical physics to show that the energy of an electron in any one of these orbits is inversely proportional to the square of the integer n .

The difference between the energies of any two orbits is therefore given by the following equation.

In this equation, n 1 and n 2 are both integers and R H is the proportionality constant known as the Rydberg constant.

Planck's equation states that the energy of a photon is proportional to its frequency.

Substituting the relationship between the frequency, wavelength, and the speed of light into this equation suggests that the energy of a photon is inversely proportional to its wavelength. The inverse of the wavelength of electromagnetic radiation is therefore directly proportional to the energy of this radiation.

By properly defining the units of the constant, R H , Bohr was able to show that the wavelengths of the light given off or absorbed by a hydrogen atom should be given by the following equation.

Thus, once he introduced his basic assumptions, Bohr was able to derive an equation that matched the relationship obtained from the analysis of the spectrum of the hydrogen atom.

According to the Bohr model, the wavelength of the light emitted by a hydrogen atom when the electron falls from a high energy ( n = 4) orbit into a lower energy ( n = 2) orbit.

Substituting the appropriate values of R H , n 1 , and n 2 into the equation shown above gives the following result.

Solving for the wavelength of this light gives a value of 486.3 nm, which agrees with the experimental value of 486.1 nm for the blue line in the visible spectrum of the hydrogen atom.

Wave-Particle Duality

The theory of wave-particle duality developed by Louis-Victor de Broglie eventually explained why the Bohr model was successful with atoms or ions that contained one electron. It also provided a basis for understanding why this model failed for more complex systems. Light acts as both a particle and a wave. In many ways light acts as a wave, with a characteristic frequency, wavelength, and amplitude. Light carries energy as if it contains discrete photons or packets of energy.

When an object behaves as a particle in motion, it has an energy proportional to its mass ( m ) and the speed with which it moves through space ( s ).

When it behaves as a wave, however, it has an energy that is proportional to its frequency:

By simultaneously assuming that an object can be both a particle and a wave, de Broglie set up the following equation.

By rearranging this equation, he derived a relationship between one of the wave-like properties of matter and one of its properties as a particle.

As noted in the previous section, the product of the mass of an object times the speed with which it moves is the momentum ( p ) of the particle. Thus, the de Broglie equation suggests that the wavelength ( l ) of any object in motion is inversely proportional to its momentum.

De Broglie concluded that most particles are too heavy to observe their wave properties. When the mass of an object is very small, however, the wave properties can be detected experimentally. De Broglie predicted that the mass of an electron was small enough to exhibit the properties of both particles and waves. In 1927 this prediction was confirmed when the diffraction of electrons was observed experimentally by C. J. Davisson.

De Broglie applied his theory of wave-particle duality to the Bohr model to explain why only certain orbits are allowed for the electron. He argued that only certain orbits allow the electron to satisfy both its particle and wave properties at the same time because only certain orbits have a circumference that is an integral multiple of the wavelength of the electron, as shown in the figure below.

The Bohr Model vs. Reality

At first glance, the Bohr model looks like a two-dimensional model of the atom because it restricts the motion of the electron to a circular orbit in a two-dimensional plane. In reality the Bohr model is a one-dimensional model, because a circle can be defined by specifying only one dimension: its radius, r . As a result, only one coordinate ( n ) is needed to describe the orbits in the Bohr model.

Unfortunately, electrons aren't particles that can be restricted to a one-dimensional circular orbit. They act to some extent as waves and therefore exist in three-dimensional space. The Bohr model works for one-electron atoms or ions only because certain factors present in more complex atoms are not present in these atoms or ions. To construct a model that describes the distribution of electrons in atoms that contain more than one electron we have to allow the electrons to occupy three-dimensional space. We therefore need a model that uses three coordinates to describe the distribution of electrons in these atoms.

Wave Functions and Orbitals

We still talk about the Bohr model of the atom even if the only thing this model can do is explain the spectrum of the hydrogen atom because it was the last model of the atom for which a simple physical picture can be constructed. It is easy to imagine an atom that consists of solid electrons revolving around the nucleus in circular orbits.

Erwin Schrödinger combined the equations for the behavior of waves with the de Broglie equation to generate a mathematical model for the distribution of electrons in an atom. The advantage of this model is that it consists of mathematical equations known as wave functions that satisfy the requirements placed on the behavior of electrons. The disadvantage is that it is difficult to imagine a physical model of electrons as waves.

The Schrödinger model assumes that the electron is a wave and tries to describe the regions in space, or orbitals , where electrons are most likely to be found. Instead of trying to tell us where the electron is at any time, the Schrödinger model describes the probability that an electron can be found in a given region of space at a given time. This model no longer tells us where the electron is; it only tells us where it might be.

Atomic emission spectra, Bohr’s atomic theory and Wave mechanical theory of the atom

by Heba Soffar · Published December 1, 2017 · Updated May 5, 2024

On heating atoms of pure elements – in a gaseous or vapor state – to a high temperature or exposing them to low pressure in an electrical discharge tube, they emit a radiation called emission spectrum ( line spectrum ).

Atomic emission spectra

On examining this radiant light with a device called a spectroscope, it was found that it is composed of a limited number of restricted colored lines separated by dark areas, So, it is called a line spectrum, It is worth mentioning that the physicists – at that time – were not able to explain this phenomenon.

A line spectrum is a type of spectrum composed of a small number of restricted coloured lines separated by dark areas, The radiant light is named a line spectrum because it is composed of a limited number of restricted coloured lines which are separated by dark areas.

Application: The line spectrum of the hydrogen atom appears (on examining) as four coloured lines separated by dark areas, It was found experimentally that the spectral lines are essential characteristics for each element because there are no two elements that have the same spectral lines.

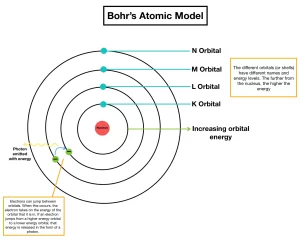
Bohr’s atomic theory

Bohr’s atomic model (1913)

The study of atomic spectra is considered the key that solved the puzzle of the atomic structure, That was the work of the Danish scientist Niels Bohr upon which he was rewarded the Nobel Prize in 1922.

Bohr’s Postulates

Points that agree with Rutherford’s postulates  

• A positively charged nucleus exists in the center of the atom .
• The number of negative electrons (revolving around the nucleus) equals the number of positive protons inside the nucleus .
• During the revolving of the electron around the nucleus, a centrifugal force a rises which is equal to the attraction force of the nucleus on the electron .

New postulates

Electrons orbit the nucleus in a rapid movement without emission or absorption of any amount of energy and the atom in this case named a stable atom.

Electrons orbit the nucleus in definite allowed energy levels, T hey can not be found at intermediate distances, at which electron moves from one energy level to another via a complete jumping.

Each electron in the atom has a definite amount of energy de pending on the distance between its energy level and the nucleus, the energy of any level increases as its radius increases, Each energy level is expressed by a whole number called the principal quantum number (n) .

When the electron acquires a quantity of energy – known a s quantum – by heating or by electric discharge, the electron jumps temporarily to a higher energy level, This is in case that the absorbed quantum of energy is equal to the difference in energies between the two levels, and the atom is known as an excited atom .

Since the electron in the excited atom is unstable, it returns to its original level with emission of the same quantum of energy (emission spectrum) in the form of radiant light that appears in the form of characteristic spectral lines of a certain wavelength and frequency.

Quantum is the amount of energy absorbed or emitted, when an electron is transferred (jumps) from one energy level to another , An Excited atom is an atom that acquires an amount o f energy by heating or by electric discharge. 

The multitude of atoms absorb different amounts of energy, and then radiate their energies producing spectral lines, These spectral lines correspond to the energy levels from which their electrons are transmitted back to the ground state.

The spectral line of the hydrogen atom does not represent the electron transferring from :

• The different energy levels to the first energy level, because the wavelength of the emitted ray from the excited electron is located in the invisible region of the ultraviolet rays.
• The seventh energy level to the second energy level, because the wavelength of the emitted ray from the excited electron is located in the invisible region of the infrared rays.

The quantum of energy required to transfer an electron between the different energy levels is not equal, One quantum is the ene rgy difference between the two energy levels.

An electron can’t move from its energy level to another if the energy absorbed or emitted is less than one quantum, (there is a half quantum for instance).

The quantum of energy required to transfer an electron between the different energy levels is not equal, because the distance and the difference in energy between them are not equal.

The quantum of energy required to transfer an electron from one energy level to another decreases as we go further from the nucleus, Because the energy gap decreases, as we go further from the nucleus.

Advantages & disadvantages of Bohr’s atomic model 

Despite of the great effort of Bohr to construct his atomic model, the quantitative calculations of his theory did not agree with all the experimental results.

Advantages (success) of Bohr’s atomic model:

• It explained the hydrogen atom spectrum.
• It introduced the idea of quantized energy to determine the electron energy in different energy levels in the atom .

Inadequacies of Bohr’s atomic model 

The most important defects o f Bohr’s theory :

• It failed to explain the spectrum of any other element, except the hydrogen atom, as it is considered the simplest electronic system which contains one electron only, even that of the helium atom contains only 2 electrons.
• It considered the electron as a negative charged particle only and ignored its wave properties.
• It postulated that it is possible to determine precisely both the location and speed of an electron at the same time, but in fact this is experimentally impossible.
• It described the electron as a particle moving in a circular planar orbit, which mean s that the hydrogen atom is planar, In fact, hydrogen atom has a spherical shape (three-dimensional coordinates).

Principle of modern atomic theory (modification of Bohr’s model):

The most important modifications are the following : 

• The dual nature of the electron .
• The Heisenberg uncertainty principle.
• The wave-mechanical theory of the atom.

Dual nature of electron 

The electron is a material particle that h as wave properties: All the previously mentioned theories considered the electron just as a minute negatively charged particle, However, all experimental data showed that the electron has a dual nature, as it is a material particle that also has wave properties.

Heisenberg uncertainty principle

Bohr’s theory postulated that it is possible to determine both the location and velocity of the electron precisely at the same time, but by applying the principles of quantum mechanics, Heisenberg concluded that the determination of both the velocity and position of an electron at the same time is practically impossible, So, to speak in terms of probability seems to be more precise, This is because the electron wave motion does not have a certain location.

Heisenberg uncertainty principle: The determination of both the velocity and position o f an electron at the same time is practically impossible and this is subjected to the laws of probability.

Wave-mechanical theory of the atom

The Austrian scientist Schrodinger (1926) applied the idea of Plank, Einstein, De Broglie and Heisenberg and could to:

• Establish the wave-mechanical theory of the atom.
• Derive a wave equation that could describe the electron wave motion in the atom .

On solving Schrodinger’s equation, it is possible to:

• Determine the allowed energy levels.
• Define the regions of space around the nucleus, where it is most probable to find the electron in each energy level.

The wave-mechanical theory changed our concept about the movement of the electron , where instead of speaking about the stable circular orbits as being completely forbidden for the electrons , the concept of the electron cloud is used to express the region of space around the nucleus.

The electron cloud is the region of space around the nucleus, in which the electron probable exists in all directions and distances (dimensions), There are regions inside the electron cloud in which the probability of finding the electron increases, each of them is termed by the orbital, Orbital is the region within the electron cloud of high probability of finding the electron .

Atoms components, Rutherford and Bohr’s Atomic Models

Evolution concept of the atomic structure, Atomic theory and Properties of cathode rays

The quantum numbers and principles of distributing electrons

Tags: atom Atomic emission spectra Atomic spectra Atomic structure Attraction force Bohr's atomic model Bohr's atomic model advantages Bohr's atomic model disadvantages Bohr's theory Centrifugal force Dual nature of electron Electric discharge Electrical discharge tube Electron cloud Electron energy Electron wave motion electrons element emission Emission spectrum Energy levels Excited atom Frequency Heating Heisenberg uncertainty principle Hydrogen atom Hydrogen atom spectrum Line spectrum mechanical Niels Bohr nucleus Protons Quantum Quantum mechanics Quantum of energy Radiant light spectra Spectral lines Spectroscope Stable atom Wave equation Wave properties Wave-mechanical theory of the atom Wavelengths

You may also like...

Alkanes economic importance, uses, physical and chemical properties

August 31, 2018

 by Heba Soffar · Published August 31, 2018 · Last modified June 21, 2024

Theories explaining the covalent bond, Octet rule and Overlapped orbitals concept

February 5, 2018

 by Heba Soffar · Published February 5, 2018 · Last modified March 6, 2024

Heat content of a substance ( Molar enthalpy ) and Thermochemical Equation

December 15, 2016

 by Heba Soffar · Published December 15, 2016 · Last modified September 21, 2019

Leave a Reply Cancel reply

You must be logged in to post a comment.

Element identification from emission spectra

Learn how to use the light analysis feature by performing an emission spectra experiment.

Scientists use models to make predictions, solve problems, and test claims. As technology improves, new evidence either confirms or contradicts previous models. Today’s atomic model is a product of centuries of refinement. In the early 1900s, Niels Bohr proposed an atomic model based on the work of many scientists, including Ernest Rutherford and Max Planck. Bohr was familiar with hydrogen’s line emission spectrum and realized the energy of emitted light must be related to an atomic structure that included energy levels. Bohr hypothesized that emitted light was the result of an electron jumping from one energy level to another. He proposed that the energy of an emitted photon is equal to the energy difference between the ground state and excited state. The distinct pattern of spectral lines unique to each atom could be related to energy levels.

Bohr’s model was critical in the development of modern atomic theory because it provided the idea that electrons are organized according to energy levels outside of an atom’s nucleus. Unfortunately, the model failed to fully explain the emission spectra of elements beyond hydrogen. The value in studying Bohr’s model lies in how it conveys a complex idea in a simple representation.

A spectrometer is a versatile tool that allows the observer to study the line emission spectrum of atoms with energized electrons. Spectrometers allow scientists to identify elements present billions of light years away from Earth, as well as provide evidence for the quantum model of the atom.

Gather materials

• PASCO Wireless Spectrometer (PS-2600A)
• Fiber Optic Cable (PS-2601)
• Multi clamp
• Gas spectrum tube
• Spectrum tube power supply

Follow these important safety precautions in addition to your regular classroom procedures:

• Wear gloves when handling the spectrum tube.
• Do NOT touch the high-voltage spectrum tube or power supply while they are active.
• Use caution around the high-voltage spectrum tube power supply at all times.

Set up the equipment

• Connect the Spectrometer to Spectrometry.
• Place the rectangular end of the fiber optic cable in the cuvette opening of the spectrometer. Align the arrows on the side of the housing so that they point in the directly of the arrow in the illustration above.
• Use a multi clamp to secure the probe or rounded end of the Fiber Optic Cable on a ring stand, as shown above.
• Place the end of the probe a distance of 2 cm or less from the gas tube. Adjust the probe to point towards the tube. Do not allow the probe to directly touch the tube.

Collect data

• Turn on the power supply.

Analyze data

Use the arrows at the bottom-right of the screen to view each reference spectrum. Which element is contained in the gas tube?

Use the wavelength of the identified peaks to solve for the energy of emitted photons using the following equation:

Questions to consider

• Which color has higher energy: red or blue?
• Is wavelength directly or indirectly related to energy?
• Is frequency directly or indirectly related to energy?

Niels Bohr used mathematical relationships to predict the structure of the atom. He assumed lower energy electrons were closest to the nucleus and higher energy electrons were farther from the nucleus.

• Suppose an electron in its ground state (n = 1) were to absorb energy and transition to an excited state (n > 1), return to ground, and release a photon as it returns to n = 1. Which path will require less energy to complete, A or B?
• Which light color is more likely to result from the path you identified as less energy: red or blue?

Astronomers use high-powered spectrometers to analyze light throughout space. How it is possible for an element to have the same line emission pattern every time it is energized, whether the element is in outer space or in a gas spectrum tube in a classroom?